Debian Project Leader Elections 2025
Time Line
| Nomination period: | Sunday 2025-03-09 00:00:00 UTC | Saturday 2025-03-15 23:59:59 UTC |
|---|---|---|
| Campaigning period: | Sunday 2025-03-16 00:00:00 UTC | Saturday 2025-04-05 23:59:59 UTC |
| Voting period: | Sunday 2025-04-06 00:00:00 UTC | Saturday 2025-04-19 23:59:59 UTC |
Please note that the new term for the project leader shall start on 2025-04-21.
Nominations
- Gianfranco Costamagna [locutusofborg@debian.org] [nomination mail] [platform]
- Julian Andres Klode [jak@debian.org] [nomination mail] [platform]
- Andreas Tille [tille@debian.org] [nomination mail] [platform]
- Sruthi Chandran [srud@debian.org] [nomination mail] [platform]
The ballot, when ready, can be requested through email by sending a signed email to ballot@vote.debian.org with the subject leader2025.
Data and Statistics
This year, like always, statistics will be gathered about ballots received and acknowledgements sent periodically during the voting period. Additionally, the list of voters will be recorded. Also, the tally sheet will also be made available to be viewed. Please remember that the project leader election has a secret ballot, so the tally sheet will not contain the voter's name but a HMAC that allows the voters to check that their vote is in the list of votes. There is a key generated for each voter that is send along with the ack for the vote.
Quorum
With the current list of voting developers, we have:
Current Developer Count = 1030
Q ( sqrt(#devel) / 2 ) = 16.0468065358812
K min(5, Q ) = 5
Quorum (3 x Q ) = 48.1404196076436
Quorum
- Option1 Reached quorum: 273 > 48.1404196076436
- Option2 Reached quorum: 264 > 48.1404196076436
- Option3 Reached quorum: 318 > 48.1404196076436
- Option4 Reached quorum: 225 > 48.1404196076436
Majority Requirement
The candidates need a simple majority to be eligible.
Majority
- Option1 passes Majority. 3.900 (273/70) > 1
- Option2 passes Majority. 3.259 (264/81) > 1
- Option3 passes Majority. 8.154 (318/39) > 1
- Option4 passes Majority. 2.009 (225/112) > 1
Outcome
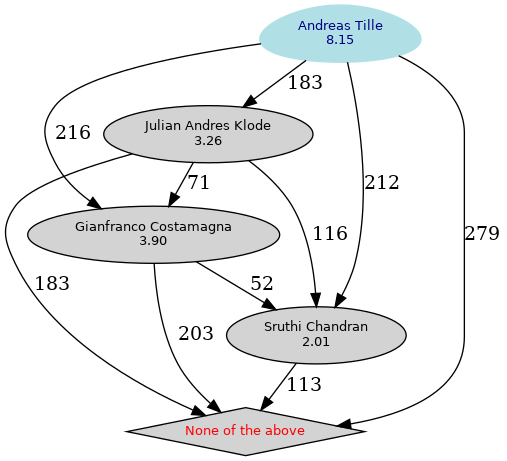
In the graph above, any pink colored nodes imply that the option did not pass majority, the Blue is the winner. The Octagon is used for the options that did not beat the default.
- Option 1 "Gianfranco Costamagna"
- Option 2 "Julian Andres Klode"
- Option 3 "Andreas Tille"
- Option 4 "Sruthi Chandran"
- Option 5 "None of the above"
In the following table, tally[row x][col y] represents the votes that option x received over option y. A more detailed explanation of the beat matrix may help in understanding the table. For understanding the Condorcet method, the Wikipedia entry is fairly informative.
| Option | |||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |
| Option 1 | 110 | 66 | 179 | 273 | |
| Option 2 | 181 | 78 | 211 | 264 | |
| Option 3 | 282 | 261 | 278 | 318 | |
| Option 4 | 127 | 95 | 66 | 225 | |
| Option 5 | 70 | 81 | 39 | 112 | |
Looking at row 2, column 1, Julian Andres Klode
received 181 votes over Gianfranco Costamagna
Looking at row 1, column 2, Gianfranco Costamagna
received 110 votes over Julian Andres Klode.
Pair-wise defeats
- Option 2 defeats Option 1 by ( 181 - 110) = 71 votes.
- Option 3 defeats Option 1 by ( 282 - 66) = 216 votes.
- Option 1 defeats Option 4 by ( 179 - 127) = 52 votes.
- Option 1 defeats Option 5 by ( 273 - 70) = 203 votes.
- Option 3 defeats Option 2 by ( 261 - 78) = 183 votes.
- Option 2 defeats Option 4 by ( 211 - 95) = 116 votes.
- Option 2 defeats Option 5 by ( 264 - 81) = 183 votes.
- Option 3 defeats Option 4 by ( 278 - 66) = 212 votes.
- Option 3 defeats Option 5 by ( 318 - 39) = 279 votes.
- Option 4 defeats Option 5 by ( 225 - 112) = 113 votes.
The Schwartz Set contains
- Option 3 "Andreas Tille"
The winners
- Option 3 "Andreas Tille"
Debian uses the Condorcet method for voting.
Simplistically, plain Condorcets method
can be stated like so :
Consider all possible two-way races between candidates.
The Condorcet winner, if there is one, is the one
candidate who can beat each other candidate in a two-way
race with that candidate.
The problem is that in complex elections, there may well
be a circular relationship in which A beats B, B beats C,
and C beats A. Most of the variations on Condorcet use
various means of resolving the tie. See
Cloneproof Schwartz Sequential Dropping
for details. Debian's variation is spelled out in the
constitution,
specifically, A.5.
Debian Project Secretary